Odds Of House Winning In Blackjack
- Odds Of House Winning In Blackjack Game
- Odds Of House Winning In Blackjack Card Game
- Odds Of House Winning In Blackjack Games
By Ion Saliu, Founder of Blackjack Mathematics
I. Fundamental Probability Issue: True Odds at Blackjack, Software Calculator
II. Fundamental Myth of Blackjack Gambling: Counting Cards
III. Theory of Streaks: Foundation of Blackjack Gambling Strategy, Systems
IV. Blackjack Resources, Software, Systems, Basic Strategy Cards (Color Charts)
1. The Fundamental Probability Issue: True Blackjack Odds - New Software Calculator
Blackjack Dealer Bust: Software to Calculate Probability, Odds, House Edge, Advantage HA. Keep this new figure in mind: The odds for a blackjack Dealer's bust are at least 33%. The bust probability is calculated by dividing the number of Dealer's busted hands to the total possible blackjack actions. The game of blackjack has a house edge of 2-3%, if the player isn’t using a strategy. This means that over time, the house is likely to win by a profit margin of 2-3% of the money you bet.
First captured by the WayBack Machine (web.archive.org) on November 21, 2002.- They say roulette is the queen of casino games. Then, blackjack is the king of the casino. Many believe that Blackjack, or 21, or twenty-one is the most popular casino game in the world. Blackjack is also the most researched game ever. It is also the only casino game with fluctuating odds (or probability). The winning chance changes with the composition of the deck. This is more about blackjack mathematics than anything else.
- For gambling is such a mathematical phenomenon that the casinos would do ... you know what to you ... if you knew well about it! This casino game is so easy to win ... but it is NOT about card counting! In truth, card counting at blackjack lopsidedly serves the greedy interests of the casinos.
- The blackjack player is honestly served only by the Fundamental Formula of Gambling (FFG). And thus the casino henchmen will threaten you if you simply write down in a notebook what you lost and what you won. Hey, that's a tax requirement in any jurisdiction!
Let me start by saying that the game of blackjack has caused me the most serious problems with casinos and gambling developers/authors/system vendors. Blackjack or Twenty-one (seen the movie 21?) is the most popular casino game and the most researched one. There are plenty of books dedicated to the so-called mathematics of blackjack.
There is worthiness in a few of such books or eBooks. For the most part, however, there isn't much mathematics in all those blackjack studies. The heart of the matter is a worthless concept known as card counting.
I do have a strong interest in blackjack. It is well documented at my website. As a matter of fact, I consider myself the best blackjack player ever. As Muhammad Ali put: 'It ain't bragging if you back it!' So, I put the money where my mouth is: I issued a casino gambling challenge, especially at the blackjack tables. So far, nobody has dared to honor my challenge. The real casino challenge is open to any gambler, gambling author, or gambling system developer — card-counting or not.
Odds Of House Winning In Blackjack Game
I wrote a book about the true mathematics of blackjack, insofar as precise probability calculations are concerned. You might be shocked to hear, but the mathematical truth is that your knowledge of blackjack probabilities or odds is dead wrong. Everything you had known was based on guesswork, albeit it educated guesswork.
To this date, the blackjack odds are the same as John Scarne calculated them in the 1950s. The computers were not the commodity they are today. And John Scarne was not a computer programmer! The way he calculated the odds made sense for the first two and three blackjack cards in a round. I quote from his 'Scarne's New Complete Guide to Gambling' (pg. 363):
- 'We find that the dealer's first two cards can produce the counts from 2 to 21 in 1,326 ways.'
Indeed, Combinations C (52, 2) = 1,326 two-card blackjack hands (combinations of 52 cards taken 2 at a time). That is the only thing... half-way mathematically correct! The truly correct method applies the mathematics of combinatorics alright. But instead of the numerical sets known as combinations, we must apply the mathematics of arrangements. The combinations represent boxed arrangements. In this case, C (52, 2) = (52 * 51) / 2 = 1,326. Arrangements A (52, 2) = (52 * 51) = 2,652 — or double the amount of combinations. Hence, we played cute and said half-true for the blackjack combinations case!
In the case of the first 2-card hands, the combinations-generating method greatly simplifies the problem at no additional cost. 10-7 is equivalent to 7-10. The problem comes to life beginning with 3-card hands. A hand like 10-6-7 is not equivalent to 10-7-6: Dealer must stop at 10-7.
- 'We'll discover that we need to know, however, and avoid most of the fractions, if we multiply 1,326 x 169 to get a common multiple of 224,094.'
Now, that's a big mystery! How did Scarne come up with that 169 factor??? Well, that's what they call an educated guess, or guesstimation! John Scarne didn't have a clue, mathematically speaking. He has never explained how he came up with that 169, kind of a new number of the beast! (There are 13 cards in each of the 4 suits in blackjack; 13 to the power of 2 equals 169... what's the relation?!)
In order to calculate the probability precisely, we must generate all the elements (blackjack hands) in lexicographical order. Nobody even knows how many hands are possible, as their size varies widely: From two cards to 10 cards (for one deck)! When two or more decks are employed, the blackjack hands can go from two cards to 11 cards.
Of course, there is a lot of blackjack software out there! But all that software belongs to the simulation category! That is, the blackjack hands are dealt randomly. Based on the well-known-by-now Ion Saliu's Paradox, random generation does not generate all possible combinations, as some elements repeat. So, we can never calculate the probability precisely based on random generation. If there are 334,490,044 total possible complete hands in blackjack, only 63% will be unique and 37% will be repeats — if we randomly generate 334,490,044 hands.
I rolled up my sleeves again. I had started years ago a blackjack project to generate all possible hands. It was very difficult. I found the project in the year of grace 2009 and also the code to generate sets from a list (last update: 2014). In this case, the list is a 52-line text file with the values of the blackjack cards, from the four 2's to the 16 Tens, to the four Aces. That's a stringent mathematical requirement. The deck of cards must be also ordered lexicographically, if we want to correctly generate all qualified sets in lexicographical order.
I generated blackjack hands as both combinations and arrangements. Then, I opened the output files (text format) and checked as many hands as possible. Yes, computing things are so much better today than just 5 years ago. The generating process is significantly faster. Also, opening large files is much easier today. My text editor of choice is my own MDIEditor And Lotto WE. It opens reasonably fast text files of several megabytes in size. The editor also uses a fixed-width font, which makes reading blackjack hands easier.
I wrote a special Web page dedicated to the topic of calculating precisely mathematically the bust-odds at blackjack following the Dealer's rules. There are lots of details, plus screenshots of the probability programs:
- Blackjack Dealer Bust: Software to Calculate Probability, Odds, House Edge, Advantage HA.
Keep this new figure in mind: The odds for a blackjack Dealer's bust are at least 33%. The bust probability is calculated by dividing the number of Dealer's busted hands to the total possible blackjack actions.Blackjack actions is a parameter that counts everything: Busted hands, pat hands (17 to 21), blackjack hands, and draws or hits to the first 2-card hands (incomplete hands). The software does NOT print the incomplete bj hands.
How can we apply the new programming to determine the bust odds for the blackjack Player? After heated debates in forums in 2014, I simply modified my software. The hit-stand limits can be set by the user. Initially, it was fixed — the ubiquitous hit all 16s and under, stand on all 17s or greater.
The software user can set the hit-limit to any value. The choices are, obviously, from 12 to 16. I tried, for example, the hit limit to 11 — that is, hit anything 11 or under, stand on anything 12 or higher. Evidently, there is no bust in such situations. That's another proof that my programming is 100% correct.
I believe that setting the hit limit to 14 or 13 reflects pretty closely the bust odds for the Player. That is, stand on 15 or greater (as arrangements):
Or, stand on 14 or greater (as arrangements):
Now, the house edge goes between something like .3355 * .2248 = 8.3% and something like .3355 * .1978 = 6.6%. It averages out to 7.5%. It is a far cry from the intentionally false house advantage (HA) of 1%, or even .5%! The overwhelming majority of blackjack players lose their bankrolls quickly, because this is NOT a 50-50 game or so much close to that margin! And always be mindful that blackjack is strongly sequential: The Dealer always plays the last hand. Otherwise, the casinos would go bankrupt!
I have seen lots of search strings in the statistics of my website related to the probability to get a blackjack (natural). This time the request was personal and directed to me:
- “In the game of blackjack determine the probability of dealing yourself a blackjack (ace face-card or ten) from a single deck. Show how you arrived at your answer. If you are not sure post an idea to get us started!”
- Oh, yes, I am very sure! As specified in this eBook, the blackjack hands can be viewed as combinations or arrangements (the order of the elements counts; like in horse racing trifectas).
1) Let's take first the combinations. There are 52 cards in one deck. There are 4 Aces and 16 face-cards and 10s. The blackjack (or natural) can occur only in the first 2 cards. We calculate first all combinations of 52 elements taken 2 at a time: C(52, 2) = (52 * 51) / 2 = 1326.
We combine now each of the 4 Aces with each of the 16 ten-valued cards: 4 * 16 = 64.
The probability to get a blackjack (natural): 64 / 1326 = .0483 = 4.83%.
2) Let's do now the calculations for arrangements. (The combinations are also considered boxed arrangements; i.e. the order of the elements does not count).
We calculate total arrangements for 52 cards taken 2 at a time: A(52, 2) = 52 * 51 = 2652.
In arrangements, the order of the cards is essential: King + Ace is distinct from Ace + King. Thus, total arrangements of 4 Aces and 16 ten-valued cards: 4 * 16 * 2 = 128.
The odds to get a blackjack (natural) as arrangements: 128 / 2652 = .0483 = 4.83%.
The generalized formula is:
Probability of a natural blackjack = (A * T) / C(R, 2)
- A = number of Aces remaining in the deck;
- T = number of 10-valued cards remaining in the deck;
- C = combination formula;
- R = total cards Remaining in the deck.
- Read a whole lot deeper analysis:
- Calculate Probability (Odds) for a Blackjack or Natural.
2. The Fundamental Myth of Blackjack Gambling: Card Counting
You might have seen that movie 21. It had absolutely no success in theaters. A DVD was released in 2008 with much more success. The 21 DVD reopened the huge gambling appetite for the so-called sure-fire strategy of counting cards at blackjack. The movie also introduced a powerfully symbolic ghost: The MIT Blackjack Team.If you watch all the features of the DVD, you will see the author of the original book that inspired the 21 movie. In his interview, the book author and the screenplay writer admitted that his book was the result of a rumor! How could people with the heads on their shoulders believe that an MIT blackjack team was even possible?! MIT (Massachusetts Institute of Technology), such a prestigious institution, would even accept the rumor of a gambling team on the premises? Let alone a real gambling team consisting of faculty and student body?! But adding MIT to a blackjack team did wonders!
The legend of card counting started with a well-written book: 'Beat the Dealer!' The author, Edward O. Thorp, was a mathematician working for IBM. He also learned computer programming in order to prove his theory on blackjack card counting.
If the player keeps track of the cards that were dealt, there will be variable situations for the player. Thorp speculated that the situations were favorable to the player when ten-valued cards and Aces (high cards) were predominant in the remainder of the card deck. Reversely, the situation was unfavorable to the player when there were more small cards (2 to 6) compared to high cards. The 7, 8, and 9-valued cards were considered neutral.
/GettyImages-200443052-001-596853f03df78c57f49b3034.jpg)
The same John Scarne we talked about before puts jokingly the advantage of card counting. Suppose there is a one-deck blackjack game with 100% penetration (i.e. all cards are dealt). The player tracked the entire deck absolutely precisely. There are 5 cards remaining in the deck: 3 eights and 2 sevens. The player would bet the maximum immediately (actually, millions if it were possible!) There is NO way the player can lose! The player would always stay on two cards (it doesn't matter if it is 7+7, or 7+8) or 8+8)! On the other hand, the dealer would always bust. It doesn't matter: 7+7 (under 17); draws an 8 and busts. Or, 8+8 (still under 17); draws a 7 and busts. Or, 7+8 = 15 (under 17); either 7 or 8 as the third card would bust the dealer's hand!
A situation like that would have occurred, but extremely rarely. To have 2 sevens and 3 eights at the bottom of a 52-card deck has a degree of certainty in the same category as the moon colliding with the earth! Keep in mind, total possible permutations of 52 cards is calculated by factorial of 52 (52! = 1 x 2 x 3 x 4 x 5 … x 50 x 51 x 52). Who can say that number?!
As a matter of fact, John Scarne challenged Edward O. Thorp to a real blackjack game in a casino. I quote from Scarne's New Complete Guide to Gambling (pg. 361):“In 1964, in an effort to test Professor Thorp's 'winning Black Jack' statements I challenged him to a $100,000 contest to be staged in Las Vegas. Thorp's reply was a big 'No'.”
This excerpt is from page 348:
- '… if he [Thorp] would like to team up with me and my partner to beat the Nevada Black Jack tables by making use of his unbeatable system. Thorp agreed and after the first three days of play in Reno, Nevada, we realized that Thorp knew nothing about the science of Black Jack play, and his countdown system never seemed to work… Thorp later admitted to us that he never really gambled…”
There is one more notable name to be mentioned here: Ken Uston. He became really famous when he appeared on CBS's newsmagazine 60 Minutes. The curiosity of the TV news division was triggered by a successful lawsuit that Ken Uston had won. He charged that he was barred from playing blackjack because of his skills as a blackjack card counter. The court system decided that no player may be discriminated against based on the skills of the player.
The curious thing is that Ken Uston became a spokesperson for the casino he had filed lawsuit against: Resorts. He appeared in TV commercials aired in New York and other big cities of the eastern board of the U.S. Even more curiously, the 60 Minutes Ken Uston segment was shot inside the same casino! Moreover, Ken Uston was allowed to win big money, as if were a fictional movie! Meanwhile, if a regular casino patron tries to take a benign photo on the premises, he/she might as well be thrown in jail! Not to mention that doing card counting can result in harassment and eviction by force, sometimes!
Card counting, as devised by Edward E. Thorp is a footnote to gambling history now. It offered a slight advantage in one-deck games, and especially towards the end of the deck. Ideally, a player could destroy the blackjack game if knowing the composition of the deck and the sequence of the remaining cards in the deck. The latter part is the real problem: Nobody will ever be able to know the sequence of the remaining cards in the deck. The count may be +5, but often the sequence is Low card, High card, Low, Low, High, High, Low, Low, High, etc. The dealer has the same probability to get the high cards. It is even more complicated when one considers that there are several blackjack players at the table.
The cards, Low and High, will be distributed randomly among them. What makes a particular player believe he/she would be the one to get the High cards? In one-deck games, and only playing head-to-head against the dealer, there is a slightly higher chance for the player to get a blackjack. The dealer also has an equally higher chance to get a blackjack. The difference is the player is paid 150% for a blackjack, while the dealer always gets 1 to 1 for a natural. Let's say the player has a 2% higher probability to get a blackjack (for a positive count, including Aces).
Under normal circumstances (count = 0, neutral), the probability of a blackjack is just under 5%. What is 2% of 5%? 0.02 x 0.05 = 0.001; that is, 0.1% better probability! That is, a 4.78% chance to get a blackjack, compared to 4.77%. Applying the Fundamental Formula of Gambling to p=4.77% and p=4.78%, we get, approximately, the same median: 14.18 and 14.15 hands. On the average, there will be 14 or 15 hands between blackjacks, in neutral counts, as well as in positive counts.
I have one problem with the two blackjack authors and researchers, Edward E. Thorp and Ken Uston. They reached a point of worshipping the method/system of card counting. I am sure they did a large number of computer simulations. I am sure they noticed the negligible effect of the system. Yet, they continued to influence a large number of potential casino players that card counting represented the road to the riches.
I would like to present a few more excerpts on Thorp and Uston. Carl Sifakis writes in his 1990 Encyclopedia of Gambling (pg. 36-37):
- “Dr. Thorp is still in computerized mathematical research, but he is now concerned with looking for values in stocks… The late Ken Uston, author of numerous books on counting, was at the end of his life involved in computer work in the Middle East, helping Kuwait track billions of dollars in investments. He was not playing blackjack in Atlantic City, although he had won a court case that barred casinos in New Jersey from refusing to let counters play. In fact, Uston, upon winning that case, didn't hit the blackjack tables in Atlantic City but signed up to do TV commercials for Resorts International, the very target of his suit… One long time gambler, Murray Friedkin, says of Thorp in 'Big Julie of Vegas' by Edward Linn: 'Thorp is the smartest man in the world; if you don't believe me, ask him…Whatever Thorp may say, I can tell you that if he has made any money on blackjack he made it by writing a book.”
Why this aura of legend surrounding card counting at blackjack? Even more mystique is added when considering that Las Vegas is still barring card counters from playing blackjack. Says Carl Sifakis:
- “And what of the casinos today? Blackjack is a much bigger game today than it was before 'Beat the Dealer' appeared. More people than ever patronize the tables and casinos today make more money from the game than ever before. That's a significant bottom line.”
- “After all, casinos make most of the money they make at the table games via blackjack. It's great advertising when someone reports a big win at the blackjack tables. Players have been encouraged for many years to believe that blackjack can be beaten, and the casinos don't want to do anything to disrupt that message.” (page 30).
Indeed, the legend of blackjack card counting plays as the most successful means of advertising for the casinos. It certainly attracts a large number of players who believe counting at blackjack is a road to riches. There are also other ways that the card counting legend favors the casinos. Read any blackjack book on card counting and virtually all of them contain the same cliché. “If you are a card counter, make some bonehead plays so that the pit boss won't ban you!” I think many card counters take the advice seriously. They do make bonehead, stupid plays from time to time just to hide their card-counting skills. What a stupid strategy for the blackjack player! What a profitable play for the casino!
It is admitted that counting cards offers no more than a 2% advantage for the blackjack player. That's a slim margin by all standards. Making bonehead plays can easily wipe out the slim, potential 2% advantage. The casinos owe big time to all authors of card-counting books. Then, in places where it is legal to ban skilled players from the blackjack action, the casinos commit downright robbery.
From what I have read, the casinos show a strong bias towards barring a blackjack player when he/she is at a serious loss! I read once that a known card counter was losing some $14,000. Exactly at that point, the pit boss approached the counter and asked him to leave the blackjack game! Get it? The $14,000 went immediately to the casino bottom line. Meanwhile, the player was deprived of a reasonable chance to recuperate his loss. After all, they say, blackjack is almost an even-odds game for a player using the basic strategy. It is fair to expect swings in the winning and losing columns of the player.
Of course, the banned player is allowed to play blackjack again. The pit bosses pretend to have forgotten him/her, until another significant loss for the player. The player is thrown out again! If the known counter is winning, the rationale is that a swing in the fortune will follow. Sooner or later, the player will encounter a severe loss. That's when you ask a counter to get out! What casino would be happy if a winner takes the money and run... for real?
The 21 movie was about counting cards at blackjack. It glamorized the tactic by adding intelligence to the whole scheme: the MIT factor! The movie was shot inside the MGM Grand casino! Surprise, surprise! Would you read again the threatening message from the chairman of the same casino (2001)? He threatened my guests that the casinos would throw out casino patrons who would dare to write down roulette numbers! Yet, the same casino allowed a big movie production inside their building! A movie production worshipping card counting...
3. Theory of Streaks: Foundation of my Blackjack Gambling Strategy
Yes, I and casino blackjack have always had a glamorous affair. I admit, I was playing blackjack at the same tables with women who showed a strong interest in me at times. A lot of mathematics is involved and implied in this casino game. Everything that has mathematics and potential to find more about Truth attracts me as pollen attracts a hard-working bee.I was a farm hand since my arrival to the United States in 1985. It was hard physical work. I always looked at the positive side as well. The physical work balanced my intense intellectual work, including computer programming. Unfortunately, hard physical work leads to physical pain more often than not. In early spring of 1996, unbearable back pain took me out of farm labor. I was unable to find employment until late summer of 1997.
One of the things I did was studying casino gambling. I used one of Thorp's terms: gambling mathematics. It was definitely mathematics. I saw also a means to make some money. I thought numerous times of turning into a professional gambler. There was a problem nonetheless: Bankroll. I barely had money just to survive. I am not ashamed of telling the truth. Besides, I made Nietzsche's philosophy come alive: ”What does not kill me makes me stronger.”
I did receive employment in the late summer of 1997. I worked mostly in temporary jobs. Good thing is I was paid. I was able to put food on the table and sleep under a roof. Additionally, I was able to save some money. I tried to build a bankroll for casino gambling.
Going to a casino was a freebie from where I live. The casinos offered free bus drives. Actually, you paid $20 and the casinos gave you your money back on arrival. They are good at mathematics, aren't they? Just about everybody loses that $20 minutes after entering the casino! Well, not me!
I hit the Atlantic City casinos a few times until the end of that year of grace 1997. I had a couple hundred dollars when I first started with blackjack. I returned home with more than the double of that amount. My window of opportunity was five hours. The bus trip would start early in the morning (Wednesday or Sunday). The trip to Atlantic City took around five hours. The trip back home would be five hours later.
The casino bosses were not mean during my first hits in 1997 - 1998. First of all, I was a small-timer: No more that 200-300 dollars per session in winnings. Besides, they might have tried to figure out my system. It was clear to them that I applied a form of Martingale betting. Most gamblers know that Martingale betting requires doubling up the bet after each loss. Well, it was a whole lot more than that and a whole lot more refined, in my case. The only negative reactions I recollect came from other gamblers.
Keep also in mind that my blackjack system was based on the wrong assumption that the dealer's bust at blackjack was 28%, as taken as a biblical figure in the gambling kingdom. As seen in another chapter, that figure is significantly higher. Therefore, the house advantage at blackjack is higher. Also importantly, the player's position at the table is relevant. At that time, I tried the middle position.
By contrast, most blackjack players prefer the last position at the table (just before the dealer). That is the best position at the blackjack table: The last seat or the third base. It is a mathematical law. The more players before me bust, the lower the degree of certainty that I will bust — the BJ dealer as well. It is worth repeating. The bust probability is constant, while the degree of certainty of serial busts decreases. It is similar to consecutive heads/tails in coin tossing. Nobody gets three or four heads in a row with the same frequency as just one heads followed by one tails.
And thusly we returned to the concept of streaks once more. As I said before, probability theory might as well be named the science of streaks. The core of my casino blackjack strategy was founded on the application of streaks. The method required reasonably accurate records of the hands I played as loss or win. When I applied my gambling strategy in writing, I noted a loss by – (a minus sign) and a win by +.
Theory of probability, in general, is the science of the streaks and skips. A statement such as 'This event will always have the probability equal to zero point zero zero three four etc.' is virtually meaningless. The probability represents the ratio of the favorable cases over total possible cases. So, it works with integers. In real-life we deal with integers (discrete values) such as numbers of elements and numbers of trials. The events will hit, or miss, in streaks pretty clearly predicted by rules and formulas of probability theory. If you play longer sessions at the blackjack table, for example, you will face a higher probability of some very long losing streaks. But play shorter blackjack sessions, and there is a far better chance that you'll escape with shorter losing streaks. It is highly recommended to play blackjack more aggressively at the beginning of short sessions. Then, move on to another table, preferably another casino.
A session at the blackjack table can be recorded as a succession of streaks. For example, from the Player's perspective –
Win Loss WW LLL Push L W...etc.
The streaks can be also viewed as skips between hits; e.g. how many hands the player skipped between two hits (wins). The underpinning of the streaks is undeniably mathematical. If you don't believe me, take Warren Weaver's word on it. Please reopen his informative book, Lady Luck — the Theory of Probability at page 346. The streaks can be calculated precisely and real events concur with the formulas.
Warren Weaver does the calculations by brute force. He takes the simplest and most fair game of chance: Coin tossing. Let's say we consider a total of 1024 tosses (it 2 to the power of 10; easier to work with, as the probability is 1/2). How many 4 consecutive runs of heads (H) can we toss in 1024 trials? Evidently, 4 heads should appear exactly in this pattern: THHHHT (where T stands for tails). The combined probability of that pattern is: 1/2 x {1/2 x 1/2 x 1/2 x 1/2} x 1/2 = 1/64. If we multiply 1/64 by the total number of tosses, the result is:1/64 x 1024 = 16 runs of 4 consecutive heads (or tails, for that matter).
I wrote software to calculate the number of streaks (or runs of consecutive outcomes) of various lengths for any given probability and number of trials. The program: Streaks.
My presentation here starts with the old blackjack figure of a dealer bust equal to 28%. Many blackjack players will still believe in that predicament for some time to come. I present here only the result of discarding the pushes. The approach considers the blackjack probabilities as follows:
~ Dealer: p = 52% (or 0.52);
~ Player: p = 48% (or 0.48).
Number of hands to play: 1000 (or changing blackjack tables around 5 times a day).
Following is the total streaks for the Dealer and the Player in 1000 hands, from single streaks (1) and 2 to 8 multiple like-streaks.
The 20 extra blackjack hands are most mathematically likely allocated as 11 (52%) to the dealer and 9 (48%) to the player. Thus, the dealer can expect up to 11 consecutive wins in 1000 hands – one time; the player can expect up to 9 consecutive wins in 1000 blackjack hands - once.
The probability for dealer to bust: 28%. In a majority of cases, the blackjack dealer busts in two consecutive hands, or skipping one hand, or skipping two hands. The same is not true for the player. The basic strategy blackjack player busts 17% of the time. Therefore, the streaks of the player's bust are longer.
Let's calculate the number of streaks of losing exactly four consecutive blackjack hands (from the player's perspective). We symbolize a dealer's win by L and a player's win by W. We can calculate the number of the streaks consisting of exactly four consecutive losses for the Player.
That is, calculate the number of the streaks in the format WLLLLW. If probability for W = .48 and the probability for L = 52%, then the combined probability is: .48 * (.52 * .52 * .52 * .52) * .48 = .016845963 or 1 in 59 or 60 hands. Mathematics of gambling expects such a streak once every 60 hands, or twice in 120 blackjack hands. Or, 17 times in 1000 continuous blackjack hands. Yes, it is possible, albeit mathematically rarely, that the blackjack player can experience 3+ such streaks in 100 hands. Equivalently, however, (casino executives hate the equivalent thing!) the blackjack player could experience 100-hand sessions with all losing streaks shorter than four (3-)! Those are likely sessions with longer player's win-streaks, usually with more frequent dealer's busts!
Now, the more pessimistic reality in blackjack, from the player's perspective. I am a strong believer in the validation of any idea or theory. My latest blackjack software (2009) undeniably proves that the percentage of the bust hands is, at least, 33%. The bust hands were generated following the dealer's rule: Hit all hands under 16 and stand on all hands 17 or higher. In this case, the house advantage (HA) at blackjack becomes: 33.6% x 33.6% = 11.5%. That HA figure is valid only for one player against the dealer. If we deduct the traditional 4% gained by the basic strategy player, we reach a 7.5% house advantage at blackjack.
The streaks will be worse for the player under these new circumstances (which I believe to be the correct ones). No wonder so many blackjack players are dismayed to hear that blackjack is almost a 50-50 game, if playing basic strategy! The players lose far more than the so-called mathematical expectation. I did hear casino players at the blackjack tables expressing anger after quickly losing all their chips. ”It was better to play roulette,” some said. I certainly agree with them right now. The house edge at roulette is better than in blackjack! Yes, some will always stigmatize you: 'Roulette is a sucker's game!'
The 33.6%-dealer-bust approach considers the blackjack probabilities as follows:
~ Dealer: p = 57% (or 0.575);
~ Player: p = 43% (or 0.425).
The number of hands to play is the same: 1000 (or changing blackjack tables around 5 times a day).
~ Dealer: 105 single-win streaks and 139 multi-win streaks;
~ Player: 140 single-win streaks and 105 multi-win streaks.
The 19 extra hands are most mathematically likely allocated as 11 (57%) to the dealer and 9 (43%) to the player. Thus, the dealer can expect up to 11 consecutive wins in 1000 hands – one time; the player can expect up to 9 consecutive wins in 1000 blackjack hands - once.
There is a significant difference that favors the blackjack dealer — that is, the casinos. A number of gamblers cautioned me with regards to John Scarne. Indeed, he helped the U.S. government with gambling matters, and especially defense against cheating the U.S. troops by gambling providers. On the other hand, he had a serious financial interest in the gambling industry. He was friends with Frank Sinatra, who, as loudly rumored, had friendship in the mob world. Perhaps John Scarne intentionally published wrong calculations pertinent to the house advantage at blackjack! The real odds would have scared away players from the most attractive and most popular casino game! Who knows?!
Noticeable differences, if playing 1000 hands continuously. My software Streaks and Blackjack always enforce the rule of playing fewer hands at the same table.
I was like most gamblers. I suspected dealer cheating quite a few times. Why does that dealer win so many hands in a row, while my wins come in single streaks that frequently? Believe me, I looked at dealers' hands so intensely sometimes that I risked being treated as hostile! I have never observed foul play, however. I played for fun in a Detroit casino in the summer of 2009. I spent some time with my daughter. One of my main points was the length of the streaks. Why did the dealer have so many long winning streaks (against me), while my winnings came mostly in the meager one hand a time? I remember asking my daughter if she remembered my streaks of 5 or longer winning hands? She was keeping track as well. I did not have a 5-streak in about 100 hands! As you see in the table above, a 5-streak of consecutive winning hands occurs 5 times in 1000 hands. That is, less than one occurrence (.5) in 100 hands.
- The blackjack dealer has undoubtedly the best position at the table. No blackjack player can ever take the dealer's position. The best position for a player is just before the dealer (the last seat or the third base). The more players at the table, the better for the player in the last seat. The more players busted before the third base, the lower the bust probability for the blackjack player in the last position. The reverse is also true: No busts by the players before you, in the last position, your bust probability is higher — the blackjack dealer's, too!
- If you play blackjack, be sure to sit in last chair, just before the dealer. The more players at the blackjack table, the better you are. And be sure to chip in with at least the table's maximum limit (to withstand long losing streaks). Try to play no more than 100 hands at the same table (higher probability for shorter losing streaks). You can play much more aggressively within the first 50-100 blackjack hands. If you and I happen to be at the same table, be grateful and let me sit at the third base! You can still learn a blackjack trick or two by watching me...
- Hear now another new bold statement: Blackjack is a positional game of chance. The position of the players, dealer included, is of the essence. The position is next organically linked to the sequence of the cards being dealt, and therefore the blackjack hands. The degree of certainty for a busted hand depends, indirectly proportionately, to the position of the player (the lexicographic index of the sequence). That's the main reason why the blackjack dealer has the best position at the table (lexicographic sequence).
- One cannot apply here the probability of the binomial distribution because of the fixed direction of the card dealing. The game would be a lot fairer if the dealing of the cards would be like in holdem poker. In poker, every player will be the first, the second, ..., the last hand, ..., and again ..., hand. The blackjack player, unfortunately, can't change the hand position or sequence... other than moving to another table...
The following passages show how I applied my streak strategy at the time when I thought the dealer bust was 28%. I also considered that player's probability was 48%, while dealer's was set to 52%. In the light of the new blackjack odds, the situation is worse for the player: 42.5% to 57.5%. In hindsight, my previous blackjack strategy was more aggressive than mathematically advised.
Yet, I was able to win consistently, up to 2003 when I reached a serious bump on the road. The floor manager of an Atlantic City casino prevented me from playing blackjack. I had been impeded to play roulette the same day, in the same casino. I did carry a pocket notebook (pencil-and-paper) and wrote down my losing and winning streaks. It was not a cheating device, by any stretch of imagination! A pencil is not an electronic device, like a miniature camera to peek at the cards in the deck! I never touched a deck of cards or a roulette wheel. I documented thoroughly the incidents and published several pages at my website especially Casinos Bar, Ban Winning Gamblers, Skilled Players.
You will find exact times and badge numbers and even names of the casino personnel involved. I didn't use a notebook in my previous casino outings. I memorized my streaks in the manner presented in detail here: The Best Casino Gambling Systems: Blackjack, Roulette, Limited Martingale Betting, Progressions.
I martingale more aggressively at the beginning of the blackjack game. I martingale the first count when it reaches 4. Since the second parameter is more consistent, I martingale it after it reaches 3. I go up to 7 in both cases (2-4-8 and 2-4-8-18). I stop at 7. My probability software Streaks proves the more aggressive betting at the beginning of a blackjack (or gambling) session. The degree of certainty DC for long streaks (losing or winning streaks) is lower.
I become more cautious after 100 blackjack hands or so. I martingale the first count after it reaches 6 (or 5 earlier in the game) and the second count after it reaches 5 (or 4 earlier in the game). I go as much as I can or allowed. If I sense that the streak is real bad, especially inside the same shoe, I stop the Martingale after 7, and start another one with 3 units after the previous streak ends.
My full system, as used with a notebook, keeps track of several streaks: Win (W), Loss (L), Dealer Bust (DB), Player Bust (PB), Dealer Blackjack (DJ), Player BJ (PJ). In my trimmed down version, without the notebook, I only keep track of two streaks: Win (W+), Loss (L-).
I recreate here a fragment with my real play at Trump Plaza Casino, July 21, 2003, beginning 5:30 PM; blackjack dealer: Ginny.
The interpretation.
Odds Of House Winning In Blackjack Card Game
As you can see, I did not go for the kill: My maximum bet was $50. The minimum bet was $10, the maximum limit at that blackjack table was $500. The 1 to 100 ratio of the bet spread is the minimum I accept. The + sign means I won; e.g. 40+ B (line #10) means I bet and won $40; the dealer busted that hand. The minus sign means I lost that hand; e.g. 25- b (line #14) means I bet and lost $25, while busting. I always differentiate between a dealer bust (B) and my busted hand (b). The j means a blackjack or natural (both for dealer or player). The session was short (under 100 blackjack hands). My longest losing streak was 5 consecutive hands. My longest winning streak was 4 consecutive hands (two occurrences). I won over $300, not counting my generous tips.
Yes, things can go wrong even in short sessions. A blackjack player might encounter a really long losing streak. As a matter of fact, that's the mantra of all casino executives or gambling gurus who have a strong financial interest (selling non-mathematical systems). They will tell you that you are going to lose in very long streaks. But they never mention the opposite: The gambler can win in very long streaks. I call that the gambler's fallacy in reverse. Things happen both ways: They are mathematical, but they represent the rare exception, not the norm. If you experience a long winning streak, my advice is to leave that blackjack table as soon as the hot streak ends.
- Axiomatic one, you can watch my streak-gambling system beating an impartial blackjack computer program simulating a continuous shuffling machine (CSM).
The casinos react with hostility to the new blackjack dealer's bust odds, probability calculations by Ion Saliu in 2009. Blackjack is a casino game far worse than assumed by most gamblers.
No, the casinos couldn't resist reacting... once again! They should know by now that I don't get intimidated. Yet, they keep reacting every time I publish something of great importance. No doubt, my new research in calculating the blackjack odds of busting is ground-breaking. The new figures will surely have an impact on the game of blackjack. They even make me a criminal by association! They compare me to the criminals who become their own lawyers! Please read an hostile email I suspect originated in a casino highly paid office: Hostile Reaction by Casinos to the New Blackjack Dealer Bust Odds, Probability Calculations.
Blackjack: Software, Content, Resources, Systems, Basic Strategy, Card Counting
See above: The comprehensive directory of the pages and materials on the subject of blackjack, baccarat, software, systems, and basic strategy.For the best mathematical casino gambling strategy (approach) for non-system players, read:
- Casinos, Gambling, Win in Casino with Mathematical Systems.
- The Best Strategy for Lottery, Gambling, Sports Betting, Horse Racing, Blackjack, Roulette.
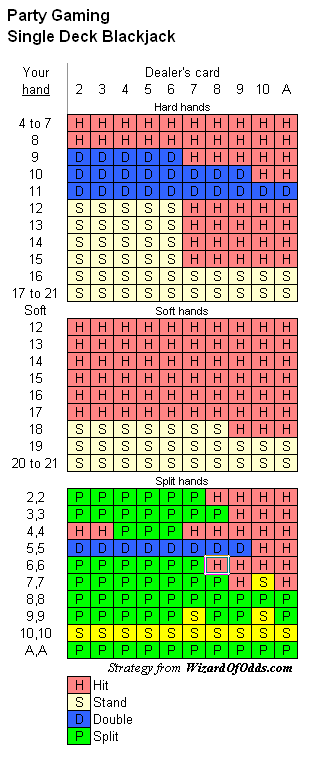
- The Best Blackjack Basic Strategy: Free Cards, Charts.
All three color-coded charts in one file, in the best decision-making sequence: Split Pairs, to Double Down, to Hit or Stand. - Gambling Mathematics in Blackjack Proves Deception of Card-Counting Systems.
- Calculate Blackjack Probability, Odds: Natural 21, Insurance, Double-Down Hands, Pairs.
- New Casino Games by Ion Saliu:Parpaluck Blackjack.
- ABC: The Best Blackjack Card-Counting System by Ion Saliu — the only system founded on mathematics and formulas.
- Reality Blackjack: Real, Fake Odds, House Advantage, Edge.
- DownloadSoftware: Casino Gambling, Roulette, Blackjack, Baccarat, Craps.
- Specific software for blackjack, BJ
~ BJAQK and Blackjack: Probability and statistical analyses of thousands of blackjack hands from the perspective of a strict blackjack old basic strategy (OBS) player.
Read Ion Saliu's first book in print: Probability Theory, Live!
~ Founded on valuable mathematical discoveries with a wide range of scientific applications, including probability theory applied to blackjack gambling, software, systems, new odds.
Home Search New Writings Odds, Generator Contents Forums Sitemap